NOTE: Due to a system migration the mathematical equations within the following article are not currently functioning. I will endeavour to repair this as soon as possible
Recently I have been reading through a newer edition of Young & Freedman’s University Physics, the main course textbook when I was a physics undergrad. Chapter 2 contains the following basic problem concerning 1-dimensional motion with constant acceleration. Since the book does not contain an answer or solution I decided to post a solution here. I highly recommend this book for undergraduate physics students and anyone who wants an excellent overview of the subject.
Question:
The Green Lantern is standing at the top of a tall building with height h and steps off, falling freely from rest to the ground below. He falls half of the total distance in the last 1.0 seconds of his fall. What is the height of the building?

Solution:
We can start by making a sketch of the problem. I personally find it preferable to re-formulate the problem into a very general timeline, with x the displacement from the starting position, as shown below. We know that he starts with zero velocity at time zero. Halfway down the building he has travelled h/2 metres but we do not yet know how long it took or his velocity at the halfway point. Finally, at the end point we know that the time is equal to the time at the halfway point plus one second, the total displacement is h. We do not yet know the final velocity. The only force acting on the falling object is gravity so we have constant accleration of g = 9.8 metres per second squared.
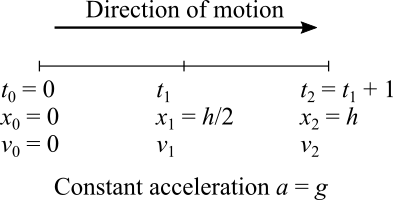
First half of fall:
We have the following equation for motion with constant acceleration (see the text book for details):
Substituting the known values for , , and :
Second half of fall:
Now for the second half of the fall: Another equation of motion derived in the text book is
Substituting known values gives us:
Subtracting h/2 from both sides and tidying up:
Combining by substitution:
We can then substitute our expression for :
We now have an equation with only one unknown, for h (remember that the acceleration due to gravity, g, is known). This is almost in quadratic form if we recognize that it is equal to
If we substitute
then we have a quadratic equation be rearranging so that
and we can solve for using the well-known equation:
where , and .
This means that
The quadratic equation gives us two possibilities for the height of the building. The trick here is to recognize that, not only is it not possible for the height of a building to be negative, but it is also not possible for the square root of the height of a building to be negative! So the only possible value for h ends up being
The quadratic equation gives us two possibilities for the total displacement h. The trick here is to recognize that, since it wouldn’t make sense for the final displacement to be negative, it also wouldn’t make sense for the square root of the final displacement to be negative. We clearly defined the acceleration to be in the positive direction and since we are starting from zero velocity we cannot end up with negative displacement! So the only valid value for h ends up being